Assignmet Mixed Strategies

Last Updated on 28. April 2023 by Martin Schuster

Task

Suppose we have the following pay-off matrix.

1. Find all Nash-equlibria in pure strategies
2. Find the equilibrium in mixed strategies.

Solution

1. {Theater; Theater} and {Football, Football}
2. T_A=0.2941, T_B=0.70588

Task

Suppose we have the following situation. In a penalty shot in football, the goalkeeper can either jump to the right or left. The player can either kick to the right or the left. The pay-off matrix looks like follows:

1. Find the Nash-equilibrium in pure strategies.
2. Find the equilibria in mixed strategies for player and goal keeper.

Solution

1. There is no Nash-equlibrium in pure strategies
2. L_S = 0,5, L_T=0,5

Task

1. Find the Nash-equilibrium in pure strategies.
2. Find the equilibria in mixed strategies for player A and player B.

Solution

1. Es gibt keines
2. x_A=0,8  I_B=0,8

Task Rock-Paper-Scissors

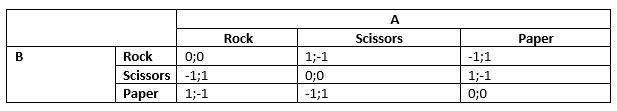
1. Find the equilibria in mixed strategies for player A and player B.

Solution

R_A = S_A = P_A = 1/3

R_B = S_B = P_B = 1/3

Task Matching Pennies

Calculate the equilibrium in mixed strategies for father and son.

Solution

H_s = 2/3

H_F = 2/3