Prisoner’s Dilemma

One of the most prominent games in game theory is the prisoner’s dilemma. We include this as a topic because it is a very important finding in the game theory.

The game illustrates that individually rational behavior doesn’t always lead to a collectively (and eventually individually) favorable result.

The cover story goes like that. Two suspects are alleged of a crime. The police interrogates both suspects separately but has no proof of their guilt. The only hope the police has, is that they incriminate the other one.

The only bargaining chip the police has is the evidence of a petty crime. The punishment for this petty crime is 1 year in prison. If one of the criminals confesses, it will not be punished for the petty crime and can go home. But at the same time, the other criminal is sentenced to 11 years of prison (1 year for petty crime and 10 for the major crime). If both criminals confess, they will not be punished for the petty crime but for the major crime.

The pay-off matrix looks as follows:

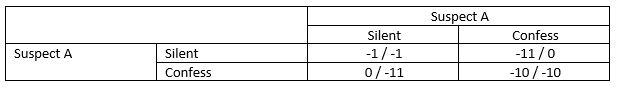
The Nash-Equilibrium is in {Confess, Confess}. Individually, it is beneficial to confess, no matter what the other suspect does. Hence, it is the dominant strategy. But if both confess, they receive a punishment of 10 years in prison each. If they had remained silent, they would only serve 1 year in prison.

The prisoner’s dilemma is a fundamental problem that cryptocurrency needs to solve. For individuals, it is beneficial not to follow the rules exactly. If only one participant does this, this single cheater is better off at the cost of the honest ones. But since it is a dominant strategy for all participants to cheat, the whole currency would become useless, and all participants would suffer a loss.