Outside Option in Game Theory

Let’s consider the following game, which is known as stag hunt. There are two hunters that can either cooperate and hunt a stag, which would bring them a pay-off of 9 each or decide to work independently and hunt a rabbit that would bring them a pay-off of 1 each. The problem is that the stag can only be hunted if both hunters cooperate. If one hunter defects, the hunter who tries to hunt the stag, receives nothing.

The following pay-off matrix shows the relevant pay-offs:

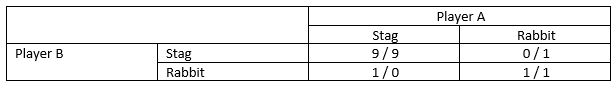
While the strategy combination {stag; stag} is the most efficient one, both players have to rely on the rationality of the other player. The first idea would be that both hunters communicate their intentions. But it is not sure if the other one can be trusted. A means to test if, let’s say player A really wants to hunt the stag, is to make the following offer:

Player A and B can independently choose a safe pay-off of 4 (or anything between 1 and 9) and not participate in the hunt. Or it can turn down this offer and participate in the hunt. If player A really wants to hunt the stag, he would turn down the safe pay-off of 4, because he hopes to get a pay-off of 9. If B also turns down the offer of 4, he also wants to hunt the stag.

If anyone of them had planned to hunt the rabbit, he would be better off accepting the pay-off of 4 instead. So, both can be sure that the other one really wants to hunt the stag instead of the rabbit. This method changes the game but could signal the commitment to a particular strategy.