Pay-off Matrix/ Bi-Matrix

A pay-off matrix is a fundamental tool in game theory. It serves as a convenient way to get an overview of the pay-offs of each player and find outcomes like Nash equilibria. We will discuss Nash equilibria in future topics.

Suppose we have two players A and B who lost themselves in a city. They can either meet at the library or at the train station. The challenge here is that they cannot communicate to coordinate their decision.

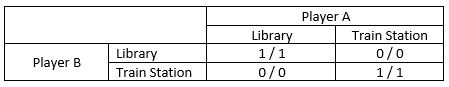
If player A goes to the library and player B goes there too, they both get a reward of 1. If they both go to the train station, they also get a reward of 1 each.

But if they miss each other (A goes to the library and B goes to the train station, or A goes to train station and B goes to library), they receive a reward of 0 each.

The following table shows the pay-offs. This kind of illustration is called a pay-off matrix or bi-matrix.

The values in the pay-off cells represent the pay-offs of the players in each situation. The values on the left in each cell tell what player B receives and the values on the right tell what player A receives.